D7 The quotient rule
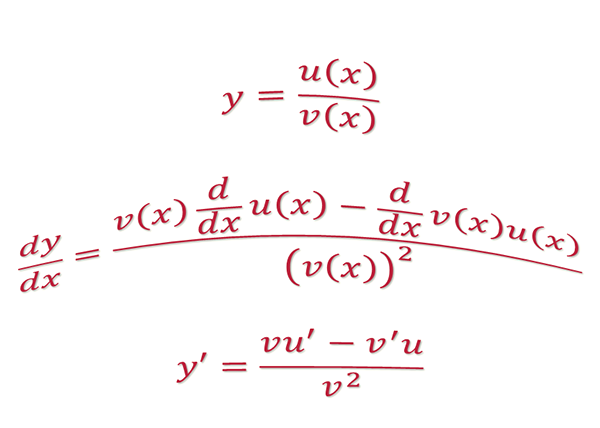
What is the quotient rule? The quotient rule is like the product rule but this time it is for one function that is divided by another (rather than multiplied).
Review this section to learn how to differentiate using the quotient rule.
Functions such as \(y=f(x)=\frac{1}{x^{2}+x}\), \(y=f(x)=\frac{\sin x}{x}\) and \(y=f(x)=\frac{x^{2}+1}{x+1}\) may be differentiated using the quotient rule.
Definition
If \[\begin{align*} f\left(x\right) & =\frac{u\left(x\right)}{v\left(x\right)} \end{align*}\] then \[\begin{align*} f'(x) & =\frac{v\left(x\right)u'(\left(x\right))-u\left(x\right)v'\left(x\right)}{\left(v\left(x\right)\right){}^{2}}. \end{align*}\] This is often abbreviated to \[\begin{align*} y' & =f'\left(x\right)\\ & =\frac{vu'-uv'}{v^{2}} \end{align*}\]
Hi, I’m Martin Lindsay from the Study and Learning Centre at RMIT University. This is a short movie on the quotient rule.
The quotient rule is used when we want to differentiate the quotient or fraction of two functions. So if f of x is equal to u of x divided by v of x then the derivative f dash x is equal to v of x times u dash x minus v dash x times u of x all divided by v of x all squared.
Now that formula is rather unwieldy to remember, so its often abbreviated to y dash is equal to v u dash minus u v dash all over v squared, and as with the product rule notice that f dash x and y dash mean the same thing.
So lets do an example. Here we want to differentiate y equals 1 plus x divided by x squared minus 3. Note first of all that we have a quotient, ie, a fraction with the numerator 1 plus x and the denominator x squared minus 3. So, we substitute, we let u equals 1 plus x, the derivative u dash is 1 and the denominator v equals x squared minus 3 from which the derivate v dash is equal to 2x. There is our formula for y dash for the quotient rule and now by substituting into that formula we get the following lines of working: so the first line was substituting, the next line we remove the brackets (be careful with the minus sign, the minus 2x squared) and finally we’ve simplified to get the answer in blue at the bottom of the slide (y dash equals minus x squared minus 2x minus 3 divided by bracket x squared minus 3 squared).
Lets do another example we want to differentiate y equals x squared divided by the log of x. Note that the log of x is the same as log of x to the base e. Again as with the previous example we have a quotient. X squared is the numerator, log of x is the denominator, so we use the quotient rule. Substituting u equals x squared, therefore u dash equals 2x and v is the log of x from which the derivative is v dash 1 over x. There is our formula for the quotient rule (y dash equals v u dash minus uv dash divided by v squared) and now substituting we get these two lines of working. Notice that when we get to the final line we need to, as before, simplify, particularly the right hand term in the numerator where one of the x’s cancels top and bottom. So that’s our answer (y dash equals 2x log of x minus x divided by bracket log x squared).
Now try some questions for yourself. The answers to these questions are on the next slide. Thanks for watching this short movie.
Examples
- If \(y=\frac{1+x}{x^{2}-3}\), find \(\frac{dy}{dx}\).
Solution
Let
\[ u=1+x\textrm{ and }v=x^{2}-3 \] then
\[ u'=1\textrm{ and }v'=2x. \] Hence using the quotient rule,
\[\begin{align*} \frac{dy}{dx} & =y'\\ & =\frac{vu'-uv'}{v^{2}}\\ & =\frac{\left(x^{2}-3\right)\left(1\right)-\left(1+x\right)2x}{\left(x^{2}-3\right){}^{2}}\\ & =\frac{x^{2}-3-2x-2x^{2}}{(x^{2}-3){}^{2}}\\ & =\frac{-x^{2}-2x-3}{(x^{2}-3){}^{2}}. \end{align*}\]
- Differentiate \(\frac{x^{2}}{\log_{e}x}\)with respect to \(x\).
Solution
Let
\[\begin{align*} y & =\frac{x^{2}}{\log_{e}\left(x\right)} \end{align*}\] and \[\begin{align*} u & =x^{2}\\ v & =\log_{e}\left(x\right). \end{align*}\] Then
\[ u'=2x\textrm{ and }v'=\frac{1}{x}. \] Hence, using the quotient rule,
\[\begin{align*} y' & =\frac{vu'-uv'}{v^{2}}\\ & =\frac{\log_{e}\left(x\right)\cdot\left(2x\right)-x^{2}\cdot\frac{1}{x}}{(\log_{e}x){}^{2}}\\ & =\frac{2x\log_{e}\left(x\right)-x}{\left(\log\left(x\right)\right){}^{2}}. \end{align*}\]
Exercise
Find the derivatives of the following functions with respect to \(x.\)
\(\quad1)\) \(f(x)=\frac{2x+1}{4x-3}\)
\(\quad2)\) \(f(x)=\frac{3}{3x^{2}+1}\)
\(\quad3)\) \(y=\frac{\sqrt{x}}{1-\sqrt{x}}\)
\(\quad4)\) \(y=\frac{e^{x}}{\sin^{2}x}\)
\(\quad1)\) \(f'(x)=\frac{-10}{\left(4x-3\right)^{2}}\)
\(\quad2)\) \(f'(x)=\frac{-18x}{\left(3x^{2}+1\right)^{2}}\)
\(\quad3)\) \(y'=\frac{1}{2x^{\frac{1}{2}}\left(1-x^{\frac{1}{2}}\right)^{2}}\) (after simplifying)
\(\quad4)\) \(y'=\frac{e^{x}(\sin x-2\cos x)}{\sin^{3}x}\) (after simplifying)
Download this page, D7 The quotient rule (PDF 166KB)
What's next... D8 Maxima and minima
