T5 Angular measurement and the unit circle
Angles are frequently measured in degrees. However, it is sometimes useful to define angles in terms of the length around the unit circle (a circle of radius = 1).
This module introduces radians as a measure of angle.
Definition of the Radian
Though angles have commonly been measured in degrees they may also be measured in units known as radians.
One radian is the angle created by bending the radius length around the arc of a circle. Consider the circle centred at the origin below. The red arc is the same length as the radius of the circle. The angle subtended at the origin \(\theta\), is one radian and is denoted by \(1^{c}\).
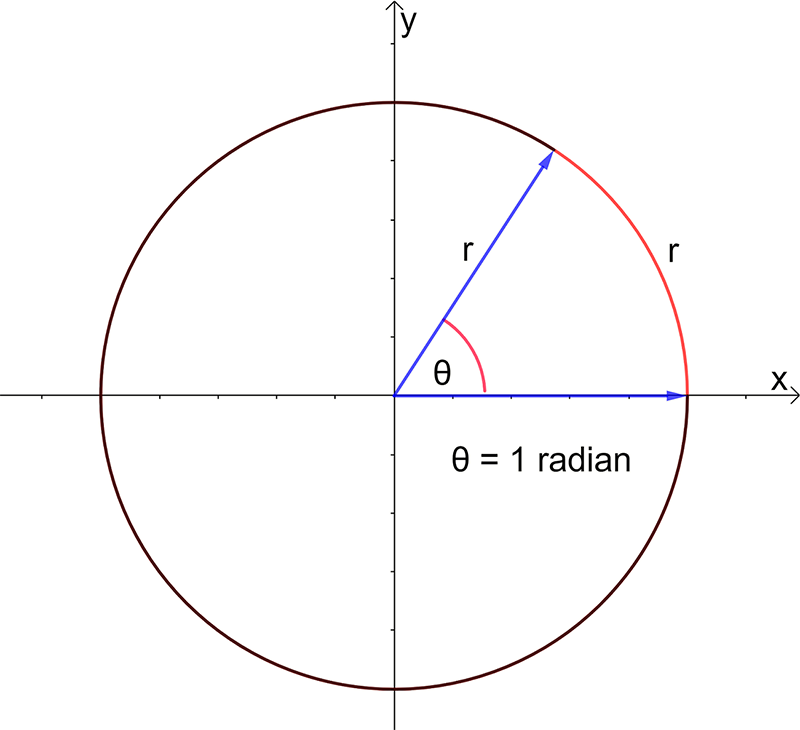
One radian is approximately \(57.3^{\circ}.\)
Converting Between Radians and Degrees
Because the circumference of a unit circle is given by the formula \(C=2\pi r\), we know \(2\pi\) radians (\(2\pi^{c}\)) is a complete rotation and the same as 360 degrees. Similarly half a rotation or 180 degrees = \(\pi\) radians (\(180^{\circ}=\pi^{c}\)).
Angles that represent fractional parts of a circle can be expressed in terms of \(\pi\).
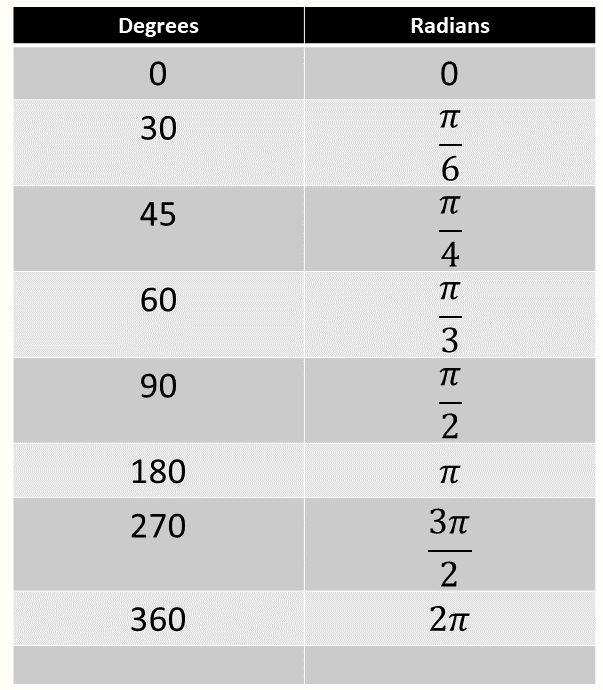
| Angle in Degrees | Angle in Radians |
|---|---|
| 90 | \(\frac{\pi}{2}\) |
| 60 | \(\frac{\pi}{3}\) |
| 45 | \(\frac{\pi}{4}\) |
| 30 | \(\frac{\pi}{6}\) |
| 270 | \(3\) \(\times90^{\circ}=3\times\frac{\pi}{2}=\frac{3\pi}{2}\) |
For other angles rearranging \(\pi^{c}=180^{\circ}\) gives: \[\begin{align*} 1^{c} & =\frac{180^{\circ}}{\pi} \end{align*}\]
and \[\begin{align*} 1^{\circ} & =\frac{\pi^{c}}{180} \end{align*}\]
Examples
- Convert \(60{{}^0}\) to radians.
\[\begin{align*} 1^{\circ} & =\frac{\pi^{c}}{180}\\ 60^{\circ} & =60\times\frac{\pi^{c}}{180}\\ 60^{\circ} & =\frac{60\pi^{c}}{180}\\ 60^{\circ} & =\frac{\pi^{c}}{3}\\ 60^{\circ} & \thickapprox\frac{3.142^{c}}{3}\\ 60^{\circ} & \thickapprox1.05^{c} \end{align*}\]
- Convert \(240{{}^0}\) to radians.
\[\begin{align*} 1^{\circ} & =\frac{\pi^{c}}{180}\\ 240^{\circ} & =240\times\frac{\pi^{c}}{180}\\ 240^{\circ} & =\frac{240\pi^{c}}{180}\\ 240^{\circ} & =\frac{4\pi^{c}}{3} \end{align*}\]
- Convert \(\dfrac{\pi}{4}\) radians to degrees.
\[\begin{align*} 1^{c} & =\frac{180^{\circ}}{\pi}\\ \frac{\pi}{4}^{c} & =\frac{\pi}{4}\times\frac{180^{\circ}}{\pi}\\ \frac{\pi}{4}^{c} & =\frac{180^{\circ}}{4}\\ \frac{\pi}{4}^{c} & =45^{\circ} \end{align*}\]
- Convert \(6.5^{\circ}\) to degrees. \[\begin{align*} 1^{c} & =\frac{180^{\circ}}{\pi}\\ 6.5^{c} & =6.5\times\frac{180^{\circ}}{\pi}\\ 6.5^{c} & \thickapprox372.4^{\circ} \end{align*}\]
Note: The symbol for radian, \(^{c}\) , is often omitted.
Exercise
- Convert the following degrees to radians
- \(30^{\circ}\quad\) b. \(270^{\circ}\quad\) c. \(20^{\circ}\quad\)d. \(450^{\circ}\quad\)e. \(135^{\circ}\quad\) f. \(57.3^{\circ}\).
- Convert the following radians to degrees
- \(\dfrac{\pi}{2}\quad\)b. \(\dfrac{5\pi}{4}\quad\) c. \(\dfrac{11\pi}{6}\quad\)d. \(3.5\pi\quad\)e. \(\pi\quad\)f. \(1\) radian.
- \(\dfrac{\pi}{6}\quad\)b. \(\dfrac{3\pi}{2}\quad\)c. \(\dfrac{\pi}{9}\)
\(\quad\quad\quad\)d. \(\dfrac{5\pi}{2}\quad\)e. \(\dfrac{3\pi}{4}\quad\)f. \(1\) radian.
- \(90^{\circ}\quad\) b. \(225^{\circ}\quad\)c. \(330^{\circ}\)
\(\quad\quad\quad\)d. \(630^{\circ}\quad\)e. \(180^{\circ}\quad\) f. \(57.3^{\circ}\).
Download this page, T5 Angular measurement and the unit circle (PDF 228KB)
What's next... T6 (FG6) Circular functions
