T3 The sine rule
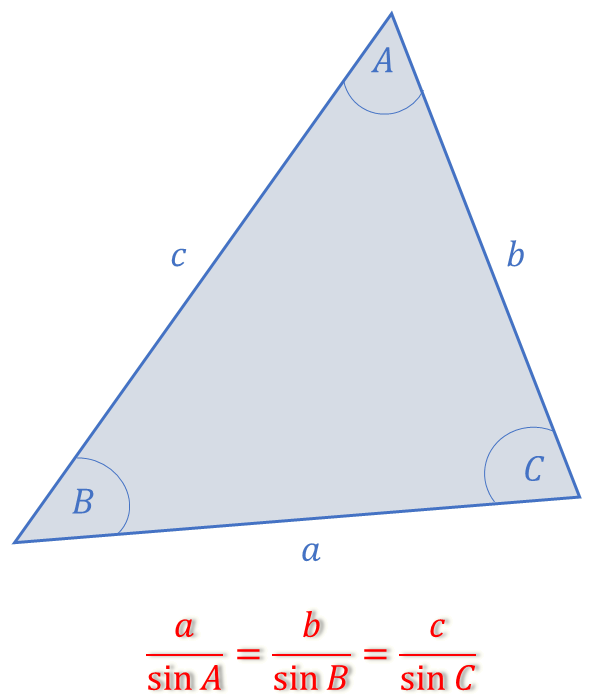
How can we apply trigonometry to triangles that do not possess a right-angle? The sine rule shows that the ratio of the length of a side, to the sine of its opposite angle, will be the same for all three sides.
The Sine rule can be used to find angles and sides in any triangle (not just a right-angled triangle) when given:
One side and any two angles or
Two sides and an angle opposite one of the given sides.
The Sine Rule
In the triangle \(ABC\) below:
angles \(A,B,C\), are the angles at the vertices \(A,B,C\) respectively
\(a,b,c\) are the side lengths opposite the angles \(A,B,C\) respectively.
The sine rule states:
\[\begin{align*} \frac{a}{\sin A} & =\frac{b}{\sin B}=\frac{c}{\sin C} \end{align*}\]
or
\[\begin{align*} \frac{\sin A}{a} & =\frac{\sin B}{b}=\frac{\sin C}{c} \end{align*}\]
Examples
- In triangle \(PQR\) find:
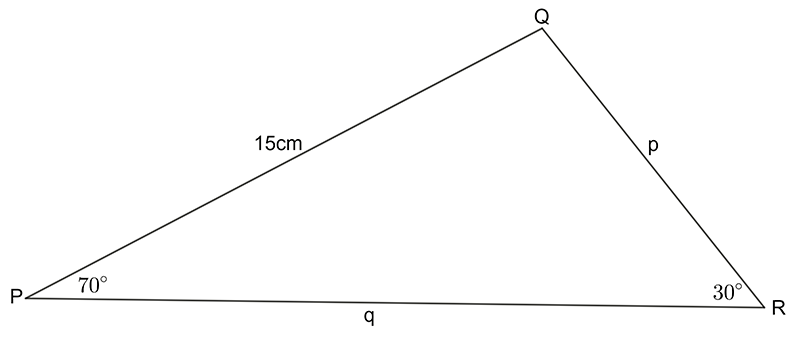
- the side length \(p\)
- the side length \(q\).
Solution a.
Use the sine rule in the form \[\begin{align*} \frac{p}{\sin P} & =\frac{r}{\sin R}\\ \frac{p}{\sin70^{\circ}} & =\frac{15}{\sin30^{\circ}}\\ p & =\frac{15\times\sin70^{\circ}}{\sin30^{\circ}}\\ p & =28.2\;. \end{align*}\] The side length is \(p=28.2\,cm.\)
Solution b)
Angle \(Q\) is found using the fact that the sum of the three interior angles of a triangle add to \(180^{\circ}\): \[\begin{align*} \angle Q & =180^{\circ}-\left(70^{\circ}+30^{\circ}\right)\\ & =80^{\circ}. \end{align*}\] From the Sine rule \[\begin{align*} \frac{q}{\sin Q} & =\frac{r}{\sin R}\\ \frac{q}{\sin80^{\circ}} & =\frac{15}{\sin30^{\circ}}\\ q & =\frac{15\times\sin80^{\circ}}{\sin30^{\circ}}\\ q & =29.6\;. \end{align*}\] The length of the side \(q=29.6\,cm.\)
- In triangle \(ABC\) find:
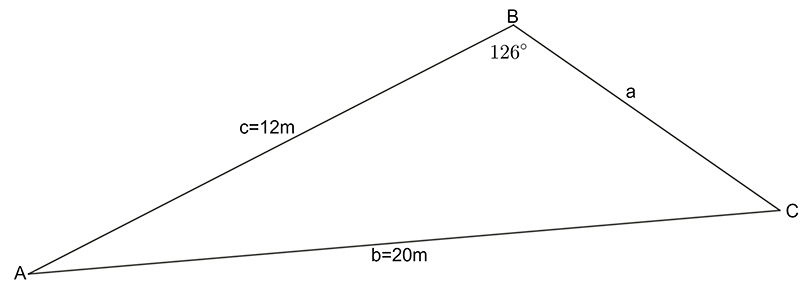
- Angle C
- Angle A
- Side length a.
Solution a.
Use the sine rule in the form \[\begin{align*} \frac{\sin A}{a} & =\frac{\sin B}{b}=\frac{\sin C}{c} \end{align*}\] The relevant part of the formula is \[\begin{align*} \frac{\sin B}{b} & =\frac{\sin C}{c}\\ \frac{\sin126^{\circ}}{20} & =\frac{\sin C}{12}\\ \frac{\sin126^{\circ}}{20}\times12 & =\sin C\\ \sin C & =0.485\\ C & =\sin^{-1}0.485\\ C & =29^{\circ}. \end{align*}\] Solution b.
\[\begin{align*} \angle A & =180^{\circ}-\left(126^{\circ}+29^{\circ}\right)\\ & =25^{\circ}. \end{align*}\] Solution c.
Use the sine rule in the form \[\begin{align*} \frac{a}{\sin A} & =\frac{b}{\sin B}=\frac{c}{\sin C} \end{align*}\] The relevant part of the formula is \[\begin{align*} \frac{a}{\sin A} & =\frac{b}{\sin B}\\ \frac{a}{\sin25^{\circ}} & =\frac{20}{\sin126^{\circ}}\\ a & =\frac{20}{\sin126^{\circ}}\times\sin25^{\circ}\\ a & =10.4m \end{align*}\]
- Given a a triangle \(ABC\) with angle \(A=30^{\circ}\), side \(c=12\) cm and side \(a=8\) cm, find angle \(C\).
Solution:
In this case there are two possible solutions as shown below.
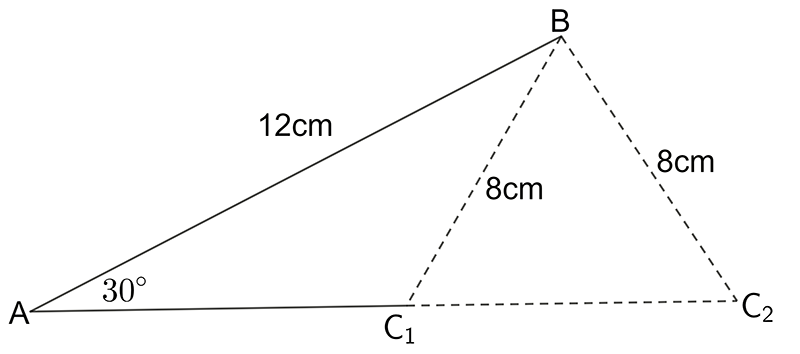
This is called the ambiguous case of the sine rule.
To solve the problem, use the sine rule in the form: \[\begin{align*}
\frac{\sin A}{a} & =\frac{\sin C}{c}\\
\frac{\sin30^{\circ}}{8} & =\frac{\sin C}{12}\\
\frac{\sin30^{\circ}}{8}\times12 & =\sin C\\
\sin C & =\frac{1}{16}\times12\\
& =0.75\\
C & =\sin^{-1}\left(0.75\right)\\
& =48.6^{\circ}\textrm{or }131.4^{\circ}.
\end{align*}\] If \(C=48.6^{\circ},\) this solution gives the triangle \(ABC_{2}.\)
If \(C=131.4^{\circ}=\left(180^{\circ}-48.6^{\circ}\right)\), this solution gives the triangle \(ABC_{1}.\)
Note: In any non-right-angled triangle, where two sides and the non-included angle are given, check for the ambiguous case.
If the angle is acute and the length of the side adjacent to the angle is greater than the length of the side opposite the angle there may be 2 possible solutions.
Exercise 1
For the following triangles find the unknown sides.
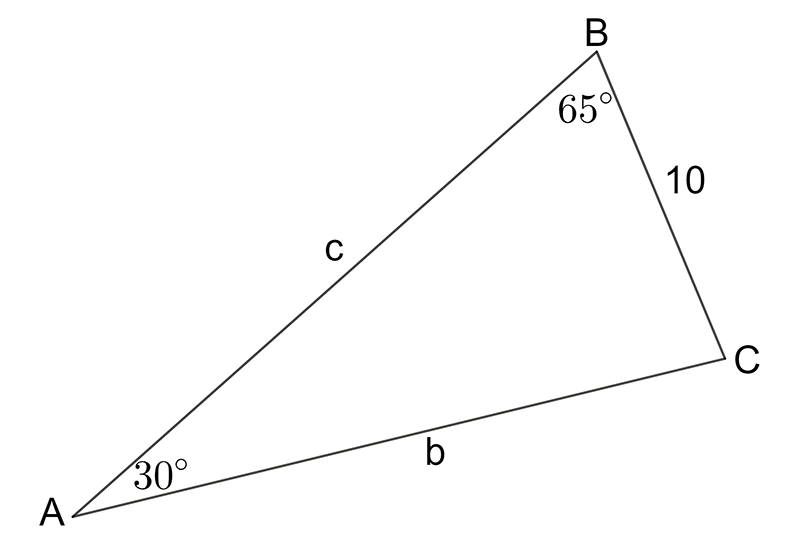
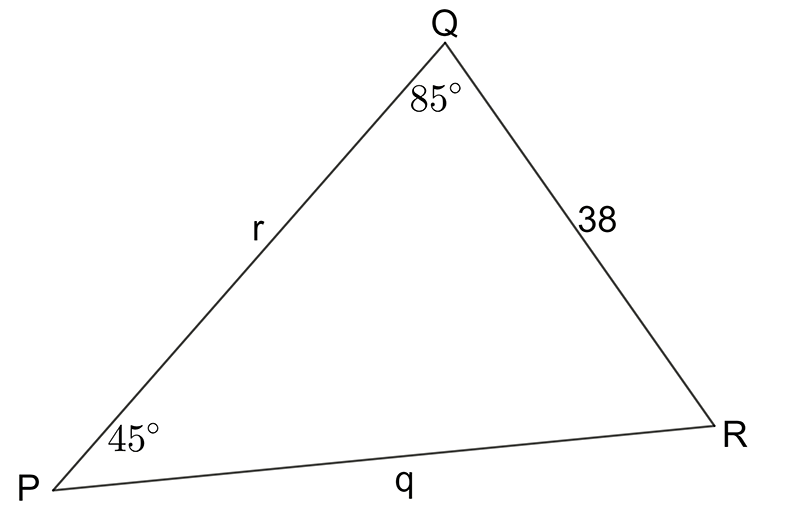
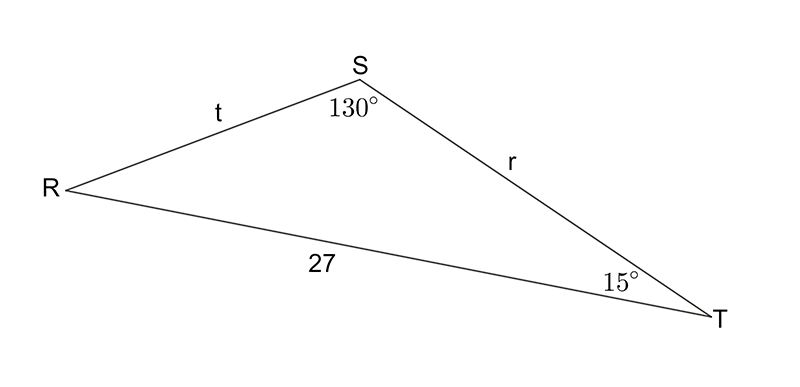
\(b=18.1\), \(c=19.9\)
\(q=53.5\), \(r=41.2\)
\(t=9.1\), \(r=20.2\)
Exercise 2
For the following triangles find all unknown angles and sides.
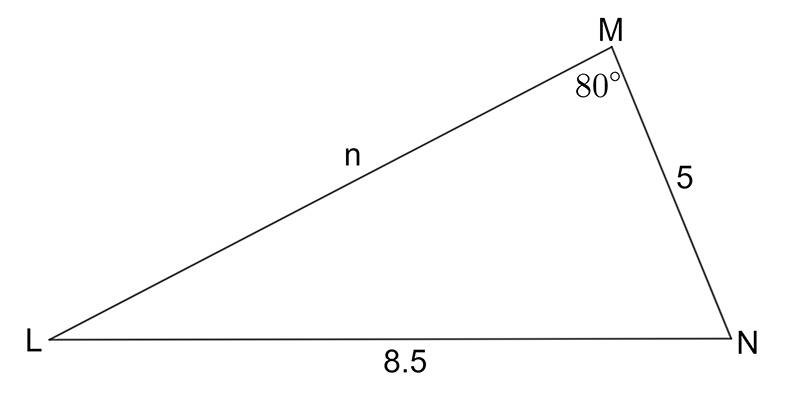
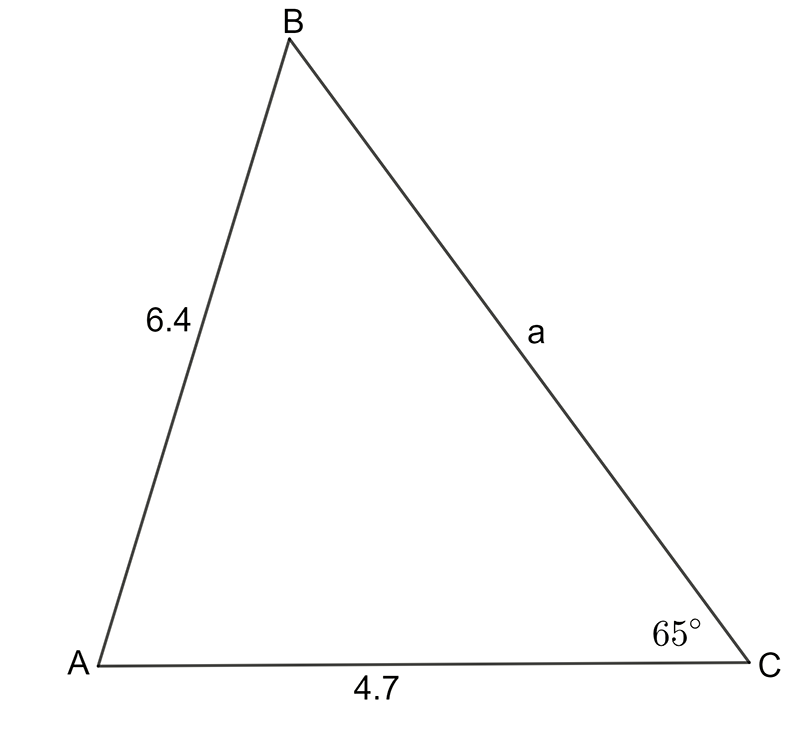
- Given triangle \(ABC\), where angle \(A=35,\) side \(a=16\) and side \(c=21,\) find the magnitude of angles \(B\) and \(C\) and the length of side \(b\).
\(L=35.4^{\circ}\) , \(N=64.6^{\circ}\), \(n=7.8\)
\(B=41.7^{\circ}\), \(A=73.3^{\circ}\), \(a=6.8\)
Ambiguous case: \(C=131.2^{\circ}\), \(B=13.8^{\circ}\), \(b=6.7\) or \(C=48.8\), \(B=96.2\), \(b=27.7\)
Download this page, T3 The sine rule (PDF 782KB)
What's next... T4 Cosine rule
