A1.5 Algebraic fractions: Multiplication and division
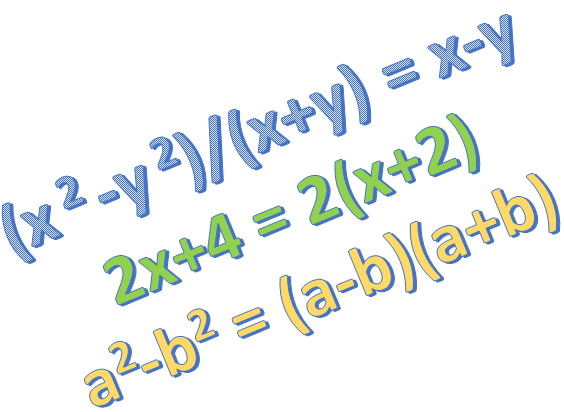
What is an algebraic fraction? The numerator(top) or denominator(bottom) of a fraction can be in algebraic form involving numbers and variables (represented by pronumerals or letters).
Hi, this is Martin Lindsay from the Study and Learning Centre at RMIT University. This is a short movie on multiplying and dividing algebraic fractions. Let’s start by looking at a normal fraction, 18 over 24. Notice that 18 and 24 are divisible by six, six into 18 goes three, sixes into 24 goes four, in other words the answer is three over four. See that six is a common factor of 18 and 24. Similarly 120 over 70 has a common factor of 10 because 10 will divide into both 120 and 70 leaving us with an answer of 12 over seven.
Here’s an algebraic fraction. Notice how I’ve ... N minus N squared becomes N minus N minus N and in doing so the factor N minus N will cancel both top and bottom. Four eight squared Y over six Y squared, notice here how I’ve expanded the X squared and the Y squared to look for factors, here there’s a factor of Y top and bottom and there’s a factor of two that divides both into four and six. And finally six X plus nine Y in the denominator will actually factorise into three brackets two X plus Y three Y and in doing so the threes now become factors top and bottom.
Now let’s multiply algebraic fractions. Here’s a simple fraction where I’ve looked for factors both top and bottom, once that’s done you can cancel the factors in the numerator with factors in the denominator, as done with the previous slide. Similarly with an algebraic fraction the As will cancel because they are common factors, there’s also a factor of seven.
Here’s a more complicated algebraic fraction, but notice the method is still the same, X minus two is a factor, X is a factor and likewise with the second example, I’ve taken a factor of three out of three M plus 12 giving me three brackets M plus four, the M squared plus four M will factorise into M brackets M plus four, again notice in doing this I can now factorise the top and the bottom factors M plus four.
Dividing algebraic fractions is very much the same but remember the rule, when you divide by a fraction you change the division sign to a multiplication sign and then you invert the fraction that comes afterwards. Here I’ve done this and I’ve factored the eight and 12. Always cancel where possible.
The second example I’ve done exactly the same, the factor of four is on the top and 24 on the bottom, fours into four, fours into 24 and finally here’s another example where I’ve taken a factor of two outside of two A plus four allowing me to factorise the top and bottom of the fraction, the factor here being the A plus two.
Now try some problems for yourself. The answers to these questions are on the next slide. Thanks for watching this short movie.
Simplifying Fractions
You have probably seen numerical fractions like \(\frac{18}{24}\) before. Generally we like to reduce fractions to their simplest form. This is done by dividing the numerator and denominator by the same number.1 The numerator is the number on top and the denominator is the number at the bottom of the fraction.
So \[\begin{alignat*}{1} \frac{18}{24} & =\frac{9}{12}\quad\mathrm{\mathrm{dividing}\ top\ and\ bottom\ numbers\ by\ two}\\ & =\frac{3}{4}\quad\mathrm{\mathrm{dividing}\ top\ and\ bottom\ numbers\ by\ three} \end{alignat*}\] this is the simplest form as there is no number that divides into \(3\) and \(4\). Note that this can be done in one step if you realise that \(6\) divides into \(18\) and \(24\). Then you have \[ \frac{18}{24}=\frac{3}{4}\quad\mathrm{\mathrm{dividing}\ top\ and\ bottom\ numbers\ by\ six.} \] Simplifying fractions by dividing the same number into both the numerator and denominator is called cancelling.
Simplifying Algebraic Fractions
We can use the same technique to simplify algebraic fractions as we did in simplifying numerical fractions. For example:
Simplify \(\frac{4x^{2}y}{6y^{2}}\).
\[\begin{align*} \frac{4x^{2}y}{6y^{2}} & =\frac{2x^{2}y}{3y^{2}}\quad\mathrm{\mathrm{dividing}\ top\ and\ bottom\ by\ two}\\ & =\frac{2x^{2}}{3y}\quad\mathrm{\mathrm{dividing}\ top\ and\ bottom\ by}\:y. \end{align*}\]Simplify \(\frac{a(b+2c)}{2ab}.\)
This example shows it is important to divide all terms on the top by the same number.2 Note that you cannot divide the \(2c\) on top and the \(2b\) on the bottom by \(2\). This is because all the terms on the top and bottom have to be divided by \(2\). So it would be wrong to write \[ \frac{b+2c}{2b}=\frac{b+c}{b} \] because the \(b\) on the top line was not divided by \(2\). \[\begin{alignat*}{1} \frac{a(b+2c)}{2ab} & =\frac{(b+2c)}{2b}\quad\mathrm{\mathrm{dividing}\ top\ and\ bottom\ by}\:a\\ & =\frac{b+2c}{2b}. \end{alignat*}\]Simplify \(\frac{m-n}{\left(m-n\right)^{2}}\). 3 This example uses the fact that \(\left(m-n\right)^{2}=\left(m-n\right)\left(m-n\right)\). Since \(m\) and \(n\) are just numbers, \(m-n\) is also a number and so we can divide by \(m-n\) provided that \(m\neq n\). If \(m=n\), \(m-n=0\) and we mustn’t divide anything by zero. \[\begin{alignat*}{1} \frac{m-n}{\left(m-n\right)^{2}} & =\frac{m-n}{\left(m-n\right)\left(m-n\right)}\quad\left(m\neq n\right)\\ & =\frac{1}{m-n}\quad\mathrm{\mathrm{dividing}\ top\ and\ bottom\ by}\:m-n. \end{alignat*}\]
Simplify \(\frac{3x^{2}y}{6x+9y}.\) 4 This example factorizes the denominator first. We then divide the top and bottom of the fraction by the common factor \(3\). \[\begin{alignat*}{1} \frac{3x^{2}y}{6x+9y} & =\frac{3x^{2}y}{3\left(2x+3y\right)}\quad\mathrm{\mathrm{taking\:out}\ the\;factor\;of\:3\ in\ the\:denominator}\\ & =\frac{x^{2}y}{2x+3y}\quad\mathrm{\mathrm{dividing}\ top\ and\ bottom\ by}\:3. \end{alignat*}\]
Simplify \(\frac{p-2}{\left(6p-3p^{2}\right)}.\) 5 This example uses the fact that \(p-2=-\left(2-p\right).\) \[\begin{alignat*}{1} \frac{p-2}{\left(6p-3p^{2}\right)} & =\frac{p-2}{3\left(2p-p^{2}\right)}\quad\mathrm{\mathrm{taking\:out}\ the\;factor\;of\:3\ in\ the\:denominator}\\ & =\frac{p-2}{3p\left(2-p\right)}\quad\mathrm{\mathrm{taking\:out}\ the\;factor\;of\:\mathit{p}\ in\ the\:denominator}\\ & =-\frac{p-2}{3p\left(p-2\right)}\quad\mathrm{\mathrm{using\;\mathit{p-\mathrm{2}=-\left(\mathrm{2}-p\right)}}}\\ & =-\frac{1}{3p}\quad\mathrm{\mathrm{dividing}\ top\ and\ bottom\ by}\:p-2. \end{alignat*}\]
Note that you can only divide the denominator and numerator if ALL the terms have a common factor. For example, it would be WRONG to write \[\begin{alignat*}{1} \frac{x+2}{2y} & =\frac{x+1}{y} \end{alignat*}\]
because the \(x\) in the top line has not been divided by 2.
To practice these techniques, please look at Exercise 1.
Multiplying Fractions
When we multiply two fractions, we multiply the numerators and the denominators of each fraction. For example, \[ \frac{3}{4}\times\frac{7}{5}=\frac{21}{20}. \]
It is best to simplify each fraction, if possible, before you do the multiplication. For example, \[\begin{alignat*}{1} \frac{15}{8}\times\frac{24}{35} & =\frac{15}{1}\times\frac{3}{35}\quad\mathrm{dividing\:the\;24\:in\:the\:top\:line\:and\:the\:8\:in\:the\:bottom\:line\:by\:8}\\ & =\frac{3}{1}\times\frac{3}{7}\quad\mathrm{dividing\:the\;15\:in\:the\:top\:line\:and\:the\:35\:in\:the\:bottom\:line\:by\:5}\\ & =\frac{9}{7}. \end{alignat*}\]
Multiplying Algebraic Fractions
You can also use these ideas with algebraic fractions. For example, \[\begin{alignat*}{1} \frac{5a}{7}\times\frac{14}{a} & =\frac{5}{7}\times\frac{14}{1}\quad\mathrm{dividing\:the\;5\mathit{a}\:in\:the\:top\:line\:and\:the\:\mathit{a}\:in\:the\:bottom\:line\:by\:\mathit{a}}\\ & =\frac{5}{1}\times\frac{2}{1}\quad\:\mathrm{dividing\:the\;14\:in\:the\:top\:line\:and\:the\:\mathit{7}\:in\:the\:bottom\:line\:by\:\mathit{7}}\\ & =\frac{10}{1}\\ & =10. \end{alignat*}\]
Here are some more examples:6 Note that we are showing every step in the examples below and so the solutions may appear long and complicated. You don’t have to do this. You can take as many steps as you like. As you get more familiar with algebra you will naturally use fewer steps to get a result.
Simplify \(\frac{x}{6\left(x-2\right)}\times\frac{3\left(x-2\right)}{x^{2}}\). \[\begin{alignat*}{1} \frac{x}{6\left(x-2\right)}\times\frac{3\left(x-2\right)}{x^{2}} & =\frac{x}{6}\times\frac{3}{x^{2}}\quad\mathrm{dividing\:the\:top\:and\:bottom\:lines\:by\:\mathit{x-2}}\\ & =\frac{x}{2}\times\frac{1}{x^{2}}\quad\mathrm{dividing\:the\:top\:and\:bottom\:lines\:by\:\mathit{3}}\\ & =\frac{1}{2}\times\frac{1}{x}\quad\mathrm{dividing\:the\:top\:and\:bottom\:lines\:by\:\mathit{x}}\\ & =\frac{1}{2x}. \end{alignat*}\]
Simplify \(\frac{3m+12}{10}\times\frac{5}{m^{2}+4m}.\) \[\begin{alignat*}{1} \frac{3m+12}{10}\times\frac{5}{m^{2}+4m} & =\frac{3\left(m+4\right)}{10}\times\frac{5}{m^{2}+4m}\quad\mathrm{taking\:out\:a\:factor\:of\:\mathit{3}}\\ & =\frac{3\left(m+4\right)}{10}\times\frac{5}{m\left(m+4\right)}\quad\mathrm{taking\:out\:a\:factor\:of\:\mathit{m}}\\ & =\frac{3}{10}\times\frac{5}{m}\quad\mathrm{dividing\:the\:top\:and\:bottom\:by\:\mathit{m+4}}\\ & =\frac{3}{2}\times\frac{1}{m}\quad\mathrm{dividing\:the\:top\:and\:bottom\:lines\:by\:\mathit{5}}\\ & =\frac{3}{2m}. \end{alignat*}\]
Dividing Fractions
The reciprocal of a fraction is just the fraction turned upside down. So the reciprocal of \(\frac{3}{4}\) is \(\frac{4}{3}\) and the reciprocal of \(\frac{2}{7}\) is \(\frac{7}{2}\). Dividing by a fraction is the same as multiplying by the reciprocal.7 When dividing fractions we change the divide sign to times and turn the last fraction upside down.
For example: \[\begin{alignat*}{1} \frac{5}{4}\div\frac{19}{8} & =\frac{5}{4}\times\frac{8}{19}\\ & =\frac{5}{1}\times\frac{2}{19}\\ & =\frac{10}{19}. \end{alignat*}\]
Dividing Algebraic Fractions
We use the same technique for dividing algebraic fractions as we use for dividing numerical fractions.
Examples
Simplify \(\frac{7p}{12}\div\frac{3}{8}.\) \[\begin{align*} \frac{7p}{12}\div\frac{3}{8} & =\frac{7p}{12}\times\frac{8}{3}\quad\mathrm{changing\:sign\:and\:inverting\:the\:last\:fraction}\\ & =\frac{7p}{3}\times\frac{2}{3}\quad\mathrm{dividing\:top\:and\:bottom\;by\:4}\\ & =\frac{14p}{9}. \end{align*}\]
Simplify \(\frac{m^{2}}{n}\div6m\). 8 Don’t forget that \(6m=\frac{6m}{1}\) \[\begin{align*} \frac{m^{2}}{n}\div6m & =\frac{m^{2}}{n}\div\frac{6m}{1}\\ & =\frac{m^{2}}{n}\times\frac{1}{6m}\quad\mathrm{changing\:sign\:and\:inverting\:the\:last\:fraction}\\ & =\frac{m}{n}\times\frac{1}{6}\quad\mathrm{dividing\:top\:and\:bottom\;by\:\mathit{m}}\\ & =\frac{m}{6n}. \end{align*}\]
Simplify \(\frac{4\left(x+3\right)}{9}\div\frac{24}{5x}.\) \[\begin{align*} \frac{4\left(x+3\right)}{9}\div\frac{24}{5x} & =\frac{4\left(x+3\right)}{9}\times\frac{5x}{24}\quad\mathrm{changing\:sign\:and\:inverting\:the\:last\:fraction}\\ & =\frac{\left(x+3\right)}{9}\times\frac{5x}{6}\quad\mathrm{dividing\:top\:and\:bottom\;by\:4}\\ & =\frac{5x\left(x+3\right)}{54}. \end{align*}\]
Simplify \(\frac{2a+4}{15}\div\frac{a+2}{6}.\) \[\begin{align*} \frac{2a+4}{15}\div\frac{a+2}{6} & =\frac{2a+4}{15}\times\frac{6}{a+2}\\ & =\frac{2\left(a+2\right)}{15}\times\frac{6}{a+2}\\ & =\frac{2}{15}\times\frac{6}{1}\\ & =\frac{2}{5}\times\frac{2}{1}\\ & =\frac{4}{5}. \end{align*}\]
Exercise 1
Simplify the following fractions:
\(\frac{12ab^{2}}{8bc}\)
\(\frac{5x-20}{5}\)
\(\frac{9u-18}{2u-4}\)
\(\frac{6t-9}{12-8t}\)
\(\frac{b}{b^{2}+7b}\)
\(\frac{\left(j+4\right)\left(j-4\right)}{3j+12}\)
\(\frac{2\left(5-v\right)}{3v-15}\)
\(\frac{9r^{2}-3r}{16r-48r^{2}}\)
\[\begin{array}{lllllllllllllll} & 1. & \frac{3ab}{2c} & & & 2. & x-4 & & & 3. & \frac{9}{2} & & & 4. & -\frac{3}{4}\\ & 5. & \frac{1}{b+7} & & & 6. & \frac{j-4}{3} & & & 7. & -\frac{2}{3} & & & 8. & -\frac{3}{16}\\ \end{array}\]
Exercise 2
A. Simplify
\(\frac{4}{5}\times\frac{15}{16}\)
\(\frac{4a}{3}\times\frac{9}{a}\)
\(\frac{32h^{2}}{9j}\times\frac{27j}{48h}\)
\(\frac{3d-2}{3}\times\frac{4}{3d-2}\)
\(\frac{2r+4}{3r-9}\times\frac{5r-15}{7r+14}\)
\(\frac{10p-5}{3}\times\frac{3q+3}{2p-1}\)
\(\frac{4g^{2}-6g}{8}\times\frac{3}{6g-9}\)
\(\frac{3-2y}{33y-11}\times\frac{18y^{2}-6y}{7-2y}\)
B. Simplify
\(\frac{4m-16}{m}\div\frac{8m-32}{8m}\)
\(\frac{6xy-5y^{2}}{4x+10y}\div\frac{12x^{2}-10xy}{12x+30y}\)
Exercise 2
\[\begin{array}{lllllllllllllll} & A1. & \frac{3}{4} & & & A2. & 12 & & & A3. & 2h & & & A4. & \frac{4}{3}\\ & A5. & \frac{10}{21} & & & A6. & 5\left(q+1\right) & & & A7. & \frac{g}{4} & & & A8. & \frac{6y\left(3-2y\right)}{11\left(7-2y\right)}\\ & B1. & 4 & & & B2. & \frac{3y}{2x} & & & & & & & & \\ \end{array}\]
Download this page, A1.5 Algebraic fractions: Multiplication and division (PDF 156KB)
