Learning Lab

In the hypothesis tests we have looked at so far, we have been concerned to find evidence that there has been a change in the population mean. The null and alternative hypotheses have been

\(H_{o}:\mu_{\overline{x}}=\mu\) (the sample mean is the same as the population mean allowing for chance variation)

\(H_{a}:\mu_{\overline{x}}\neq\mu\) (the sample mean is not the same as the population mean allowing for chance variation)

Such a test is looking for a change in either direction: has the population mean increased or has the population mean decreased?

One Sided Claims

For claims such as:

1. Queenslanders have a \(greater\) chance of skin cancer.

2. The drop-out rate for Business students is \(lower\ than\) the university average.

3. Drug A is \(more\ e\!f\!f\!ective\) than drug B.

4. Battery X \(lasts\ longer\) than 300 minutes.

5. Superannuation company Bib \(outper\!f\!ormed\) superannuation company Bob in 2017.

we would use a one sided hypothesis test. All the highlighted words imply change in one direction. Hypothesis tests in which we are only concerned with change in ONE direction are called one sided or one tailed tests.

The null and alternative hypotheses for one sided tests may be:

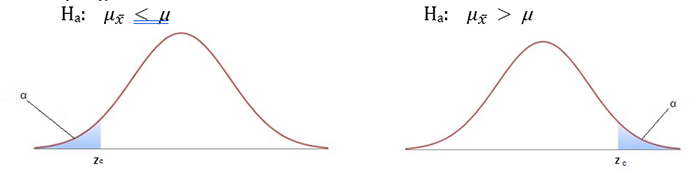
\(H_{o}:\mu_{\overline{x}}\leq\mu\) (the sample mean is not greater than the population mean after allowing for chance variation)

\(H_{a}:\mu_{\overline{x}}>\mu\) (the sample mean is greater than the population mean after allowing for chance variation)

OR

\(H_{o}:\mu_{\overline{x}}\leq\mu\) (the sample mean is not less than the population mean after allowing for chance variation)

\(H_{a}:\mu_{\overline{x}}>\mu\) (the sample mean is less than the population mean after allowing for chance variation)

Conventions on how to write one sided hypotheses vary so always check with your program.

Critical Values

In a two sided test with a significance level of \(\alpha\), there are two critical values and the area of each upper and lower rejection region is \(\frac{\alpha}{2}\).

In one sided tests with a significance level of \(\alpha\) there is only one critical value and the region of rejection with area \(\alpha\) is on either the left OR the right side of the sampling distribution

Example

A baking company claims that the amount of meat in its pies is at least \(50g\). However a consumer group has had many complaints and decides to conduct a test to check if the pies are being under filled. A random sample of \(10\) pies resulted in the following data:

\[\begin{array}{cccccccccc} 45 & 52 & 51 & 44 & 50 & 52 & 48 & 45 & 43 & 47\end{array}\]

Is there evidence in this sample that the pies are being under filled? Use \(\alpha=0.05\).

From the data: \(\overline{x}=47.7s\) and \(s=3.4\).

1. Hypotheses
   \(H_{o}:\mu_{\overline{x}}\leq50\)
   \(H_{a}:\mu_{\overline{x}}>50\)

2. Significance level
   \(\alpha=0.05\) and a one sided t-test is appropriate.

3. Critical values
   From tables: \(\alpha=0.05\), degrees of freedom \(=n-1=10-1=9\Rightarrow t_{crit}=-1.833\).

4. Test statistic:
   \(t\)= \(\frac{\overline{x}-\mu}{s_{\overline{x}}}=\frac{47-50}{\frac{3.4}{\sqrt{10}}}=-2.14\).

5. Decision
   Is the test statistic \((-2.14)\) more extreme than the critical value \((-1.833)\) ?
   Yes, therefore we reject \(H_{o}\).

6. Conclusion
   There is evidence to suggest that the pies are being filled with less than \(50g\) of meat.