FG2 Interval notation
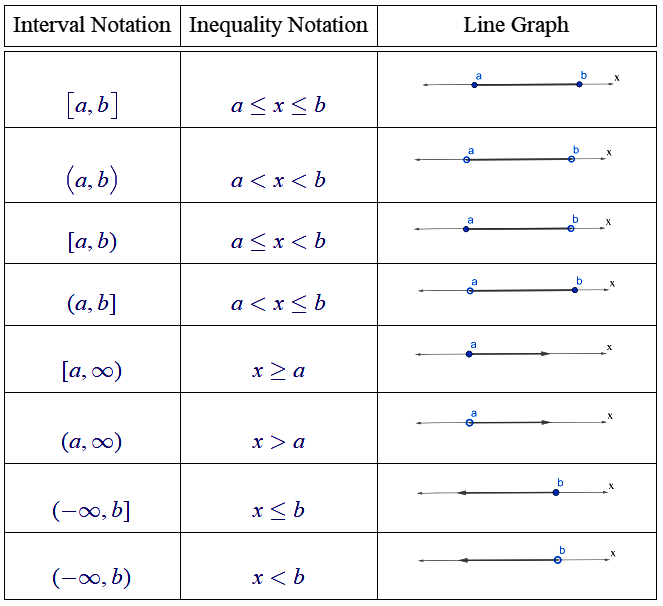
Often the domain of a function will be restricted to a subset of R. This subset is called an interval, and the end points are a and b.
Intervals
Often the domain of a function will be restricted to a subset of the set of real numbers, \(\mathbb{R}.\)
This subset is called an interval and the end points are \(a\) and \(b\) .
An interval may be represented on a real number line as follows:
In inequality notation the above number line would be written as \(a\leq x\leq b.\)
In interval notation the above interval would be written as \([a,b]\).
Closed Interval
Because the endpoints are included in the interval, this is called a closed interval and square brackets are used, eg. \(\left[2,5\right]\).
The end points on the real number line are represented by solid circles (or square brackets).
Open Interval
If the endpoints are not included in the interval, this is called an open interval and curved brackets are used, eg. \(\left(2,5\right)\).
The end points on the real number line are represented by open circles (or curved brackets).

This is written in inequality notation as \(a<x<b\) . In interval notation as \(\left(a,b\right)\).
Examples

In interval notation the smaller number is always written to the left; i.e. \([-3,5)\) not \((5,-3]\)
Note: the symbol \(\infty\) (infinity) is not a numeral.
\(\infty\) is the concept of continuing indefinitely to the right; \(-\infty\) is the concept of continuing indefinitely to the left.
Hence we cannot write \(\left[b,\infty\right]\) , \(\left[-\infty,a\right]\) or \(b\leq x\leq\infty\) etc.
Examples
- Write the following in inequality notation and graph on the real number line:
- \([-2,3)\)
Inequality notation: \(-2\leq x<3\)

- \((-\infty,3]\)
Inequality notation: \(x\leq3\)

- Write the interval notation and inequality notation for the following line graphs:

Interval notation: \((-5,6]\)
Inequality notation: \(-5<x\leq6\)

Interval notation: \([10,\infty)\)
Inequality notation: \(x\geq10\)
See Exercise 1.
Two Intervals
Two (or more) subsets of \(R\), with end points \(a\) and \(b\), and \(c\) and \(d\), respectively, can also be represented on a real number line.
Examples
- Consider the line graph below:

This is written in interval notation as \([a,b]\cup[c,d]\). The symbol \(\cup\) means “in union with”. In inequality notation this may be written: \(a\leq x\leq b\) with \(c\leq x\leq d\) , or written as \(\left\{ x:a\leq x\leq b\right\} \cup\left\{ x:c\leq x\leq d\right\}\)
- Consider the line graph below:
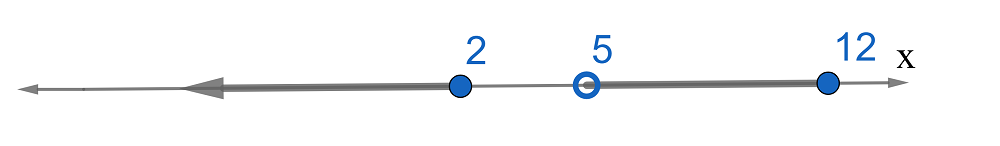
This is written in interval notation as \((-\infty,2]\cup(5,12]\). In inequality notation this may be written: \(x\leq2\) with \(5<x\leq12\) , or written as \(\left\{ x:x\leq2\right\} \cup\left\{ x:5<x\leq12\right\}\).
See Exercises 2 and 3.
Exercises
Write the following inequalities in interval notation and graph on a real number line:
(a) \(1\leq x<10\)
(b) \(-6\leq x<-4\)
(c) \(x>5\)Write the following in interval notation and inequality notation:

- Write the following in interval notation and inequality notation:

- Write the following in interval notation and inequality notation:

- Graph the following on the real number line and write in inequality notation:
(a) \(\left(-\infty,3\right)\cup(8,13]\)
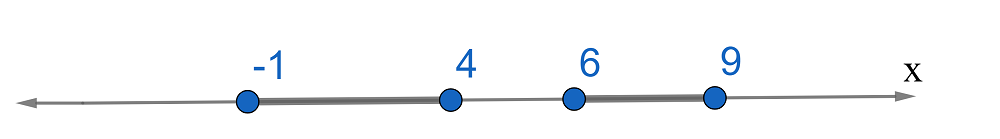
(b) \(\left[-1,4\right]\cup\left[6,9\right]\)
(c) \((-\infty,3]\cup\left(6,\infty\right)\)
- \([1,10)\)

(b) \([-6,-4)\)
(c) \((5,\infty)\)
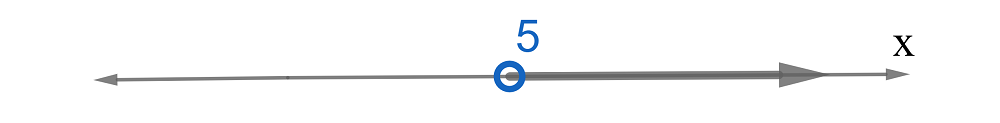
\((-\infty,5]\) ; \(x\leq5\)
\(\left(-3,0\right)\) ; \(-3<x<0\)
\([-1,4)\) ; \(-1\leq x<4\)
- \(x<3\) with \(8<x\leq13\) or \(\left\{ x:x<3\right\} \cup\left\{ x:8<x\leq13\right\}\)

- \(-1\leq x\leq4\) with \(6\leq x\leq9\) or \(\left\{ x:-1\leq x\leq4\right\} \cup\left\{ x:6\leq x\leq9\right\}\)
- \(x\leq3\) with \(x>6\) this could also be written as \(\left\{ x:x\leq3\right\} \cup\left\{ x:x>6\right\}\)

Download this page, FG2 Interval Notation (PDF 329 KB)
What's next... FG3 Inverse notation
