PF1.3 Forces: Statics
Static Equilibrium
Structures that are stable are said to be in static equilibrium. This occurs when the sum of the forces on it in each direction is zero and the sum of the torques in each direction is zero. So there are two types of static equilibrium: translational equilibrium and rotational equilibrium.
Translational Equilibrium
Translational equilibrium means that the sum of the forces (or net force) acting on the system is zero, ie, sum of the forces is zero \(\sum F\) \(=0,\)we divide all forces into horizontal and vertical components or:
Forces up = Forces down
Forces left = Forces right
Example 1
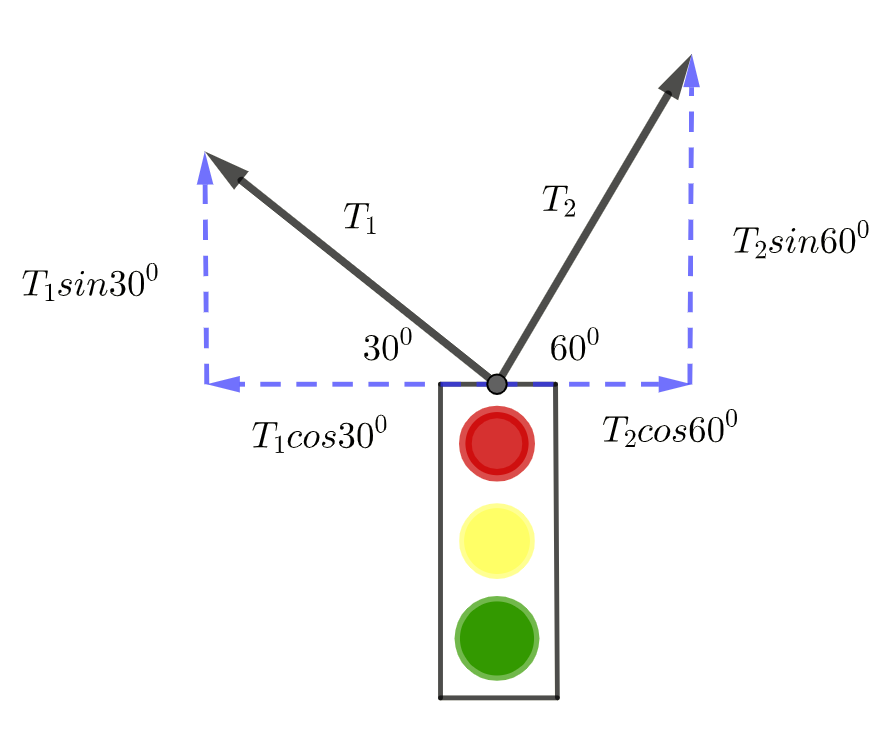
Consider a hanging set of traffic lights of mass \(m\) suspended above an intersection by a pair of wires of negligible mass, as shown below. It is easiest to break up the forces to vertical and horizontal forces.

Since this structure is in equilibrium the magnitude of the components of forces upwards must equal the magnitude of the components of forces downwards, or
Forces up = Forces down (there is no vertical motion)
Resolving \(T_{1}\) and \(T_{2}\) vertically in the diagram above:
\[\begin{align*} \textrm{forces Up} & =\textrm{forces Down}\\ T_{1}\sin30+T_{2}\sin60 & =W\\ T_{1}\sin30+T_{2}\sin60 & =mg \end{align*}\]
Alternatively, we could resolve \(T_{1}\) and \(T_{2}\) horizontally.
Forces left = Forces right (there is no horizontal motion)
Resolving \(T_{1}\) and \(T_{2}\) horizontally in the diagram above:
Note that vertical weight force W (=mg) when resolved horizontally equals zero or \(W\) \(\cos90=0\)
\[\begin{align*} \textrm{forces left} & =\textrm{forces right}\\ T_{1}\cos30^{\circ} & =T_{2}\cos60^{\circ}. \end{align*}\]
Rotational Equilibrium
Torque or Moment of a Force is the turning effect or torque \(\tau\) of a force F acting at a distance\(r\) from the axis of rotation of a rigid body is given by

\(\tau=F\times r\)
where \(F\) and \(r\) are mutually perpendicular (at right angles) to each other.
For rotational equilibrium to occur the sum of the torques (or net torque) acting on the structure is zero, ie \(\sum\tau=0\), (sum of \(\tau\) = 0) or
Clockwise Torques = Counterclockwise Torques
Example 2
Consider the seesaw example below.
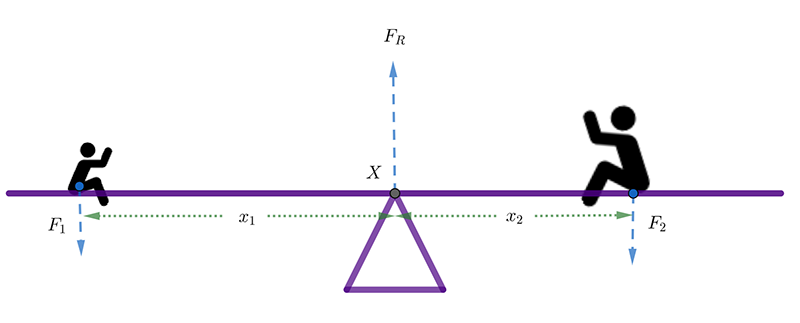
For translational equilibrium: \(\Sigma F=0\) or \[\begin{align*} \textrm{Forces Up} & =\textrm{Forces Down}\\ F_{R} & =F_{1}+F_{2} \end{align*}\]
For rotational equilibrium: \(\Sigma\tau=0\) (when a seesaw is not moving) or
\[\begin{align*} \textrm{Clockwise Torque} & =\textrm{CounterClockwise Torque}\\ F_{2}\times x_{2} & =F_{1}\times x_{1} \end{align*}\] An example of a system that is not in rotational equilibrium is a moving see-saw. If you are sitting on one end and an elephant (!) decides to sit on the other end, then it will rotate!!
Exercises
Use \(g=9.8\) \(ms^{-2}\)
- In Example 1, \(T_{1}\) \(=1000N\), and \(T_{2}\) \(=2000N\). Using vertical forces only calculate:
- the maximum weight of the traffic lights that can be supported by the cables.
- the maximum mass of the traffic lights that can be supported by the cables.
- A picture is hung as shown in the following diagram.
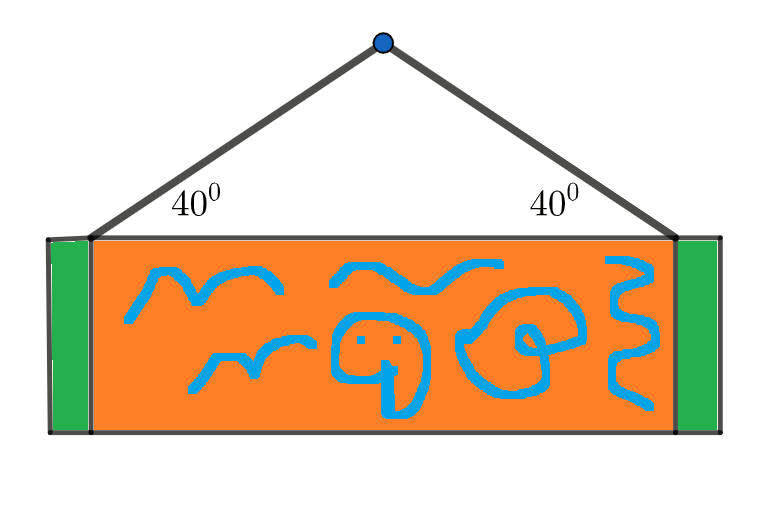
If the hanging wire has a breaking strength of \(40N\), what is the maximum possible mass of the picture?
- A \(100\,gram\) electric light is supported by two cables, one at an angle of \(60{^\circ}\) with the ceiling, and the other being perpendicular to the wall.
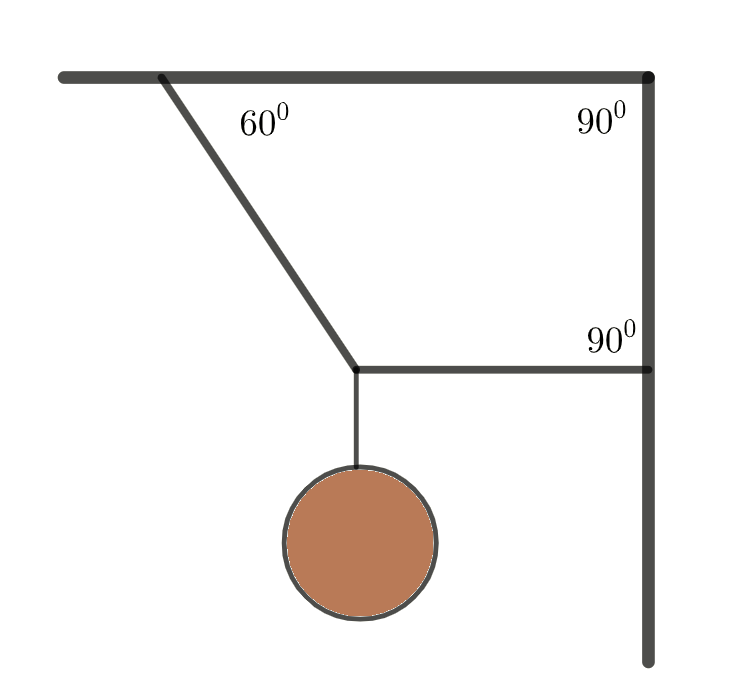
Assuming the mass of each cable is negligible, calculate the tension in each cable.
Two children are balanced on a see-saw which is supported in the middle. One child weighs \(200N\) and is \(1.2m\) from the axis, while the other child is seated \(1.5m\) from the axis. How much does the second child weigh? Ignore the mass of the see-saw.
Two children wish to make a see-saw from a \(5.0m\) plank of wood. The children weigh 25 kg and 20 kg. They each wish to sit right on the ends of the plank. Where should the plank be supported in order for it to balance?
\[\begin{array}{lll} 1a)\,T_{1}=2.2\times10^{3}\,N\,\,b)\,224\,kg & 2)\,T=5.2\,kg & 3)\,T_{1}=1.13\,N,\,T_{2}=0.57\,N\\ 4)\,W=160\,N & 5)\,2.2\,m\,\textrm{from the heavier child.} \end{array}\]
All diagrams created by RMIT
