Build an atom
Check out this PhET simulation to make your own molecule.
Atomic structure
Atomic structure underpins the behaviour of elements, their interactions, and the formation of molecules. These are fundamental to chemistry, physics, and many applied sciences. This resource explains the structure of atoms, atomic numbers and mass numbers, and isotopes and atomic masses.
The atomic model
All atoms are composed of a small, positively charged nucleus containing protons and neutrons surrounded by a larger negatively charged electron cloud. This is the atomic model, which describes the structure of atoms.
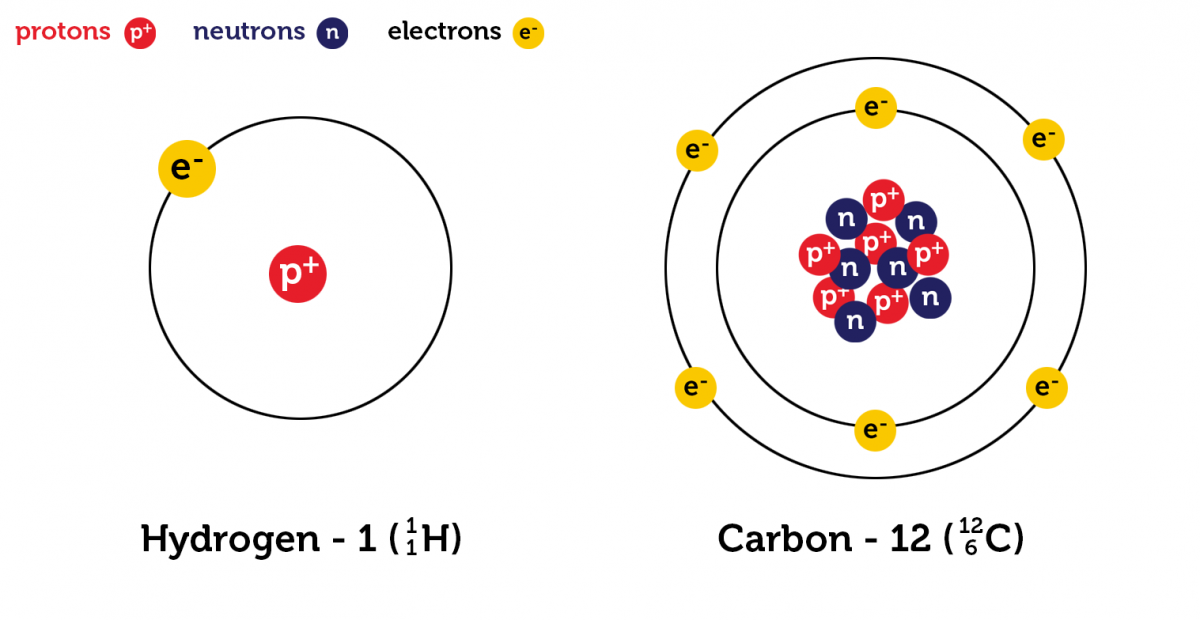
Hydrogen-1 (\(^{1}_{1}\ce{H}\)) consists of one electron, with one proton in its nucleus. Carbon-12 (\(^{12}_{6}\ce{C}\)) consists of six electrons, with six protons and six neutrons in its nucleus.
Protons, neutrons and electrons are subatomic particles. Subatomic particles present in the nucleus are known as nucleons (e.g. protons and neutrons). Properties of the subatomic particles are given in the table. You can see that protons and neutrons are almost identical in mass, whereas electrons are much smaller. Atoms are uncharged species due to the presence of an equal number of postiively charged protons and negatively charged electrons.
| Subatomic particle | Symbol | Charge | Mass (kg) | Size relative to a proton |
|---|---|---|---|---|
| proton | p | \(+1\) | \(1.673\times10^{-24}\) | \(1\) |
| neutron | n | \(0\) | \(1.675\times10^{-24}\) | \(1\) |
| electron | e | \(-1\) | \(9.109\times10^{-28}\) | \(\frac{1}{1 800}\) |
Nuclide notation
Atoms are represented using nuclide notation (also called isotope notation). This includes the chemical symbol for the element, its atomic number and mass number.
The nuclide notation of carbon-12 shows the mass number \(12\) at the top, the atomic number \(6\) at the bottom, and the chemical symbol \(\ce{C}\).
Atomic number
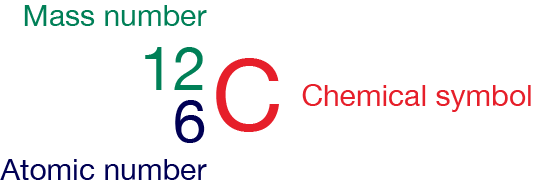
An atomic number (Z) is the number of protons present in the nucleus of an atom. Since an atom contains an equal number of protons and electrons, the atomic number also indicates the number of electrons present in an atom.
- Atomic number (Z) = Number of protons = Number of electrons
Mass number
A mass number (A) is the sum of the number of protons and neutrons present in the nucleus of an atom. It is the total number of nucleons.
- Mass number (A) = Number of protons + Number of neutrons
The number of subatomic particles present in an atom can be determined using its atomic number and mass number.
- Number of protons = Atomic number (Z)
- Number of electrons = Atomic number (Z)
- Number of neutrons = Mass number (A) – Atomic number (Z)
- Total number of subatomic particles = Mass number (A) + Atomic number (Z)
Isotopes and atomic masses
Isotopes are atoms of an element with the same number of protons and electrons, but different numbers of neutrons. Examples are:
- carbon which has three isotopes: \(\ce{_{6}^{12}C}\), \(\ce{_{6}^{13}C}\), \(\ce{_{6}^{14}C}\)
- chlorine which has two isotopes: \(\ce{_{17}^{35}Cl}\), \(\ce{_{17}^{37}Cl}\)
- hydrogen which as three isotopes: \(\ce{_{1}^{1}H}\), \(\ce{_{1}^{2}H}\), \(\ce{_{1}^{3}H}\).
Atomic mass is the weighted average mass of an atom of an element. To calculate the atomic mass, the relative percentage abundance and atomic mass of each isotope is considered. The atomic number and atomic mass are typically represented alongside the chemical symbol in the periodic table. You will learn about the periodic table later.
The carbon-12 isotope has an atomic number of \(6\), an atomic mass of \(12.01\), and the chemical symbol \(\ce{C}\).
Worked example – determining the number of subatomic particles
Calculate the number of protons, electrons and neutrons present in the atom \(\ce{_{18}^{40}Ar}\).
Step 1: Find the atomic number (Z) and mass number (A) from the nuclide notation. Z is the number written at the bottom. A is the number at the top.
\[Z=18\]
\[A=40\]
Step 2: Determine the number of protons. This is the same as the atomic number (Z).
\[\textrm{Number of protons}=Z=18\]
Step 3: Determine the number of electrons. This is the same as the atomic number (Z).
\[\textrm{Number of electrons}=Z=18\]
Step 4: Determine the number of neutrons. This is the mass number (A) minus the atomic number (Z).
\[\begin{align*}\textrm{Number of neutrons} & =A-Z\\
&=40-18\\
&=22
\end{align*}\]
The atomic number of the lithium atom is \(3\), and its mass number is \(7\). Calculate the total number of nucleons present in the atom.
Step 1: Recall that nucleons include protons and neutrons.
Step 2: Determine the number of protons. This is the same as the atomic number (Z).
\[\textrm{Number of protons}=Z=3\]
Step 3: Determine the number of neutrons. This is the mass number (A) minus the atomic number (Z).
\[\begin{align*}\textrm{Number of neutrons} & =A-Z\\
&=7-3\\
&=4
\end{align*}\]
Step 4: Calculate the number of nucleons by adding up the number of protons and neutrons.
\[\begin{align*}\textrm{Number of nucleons} & = \textrm{Number of protons}+\textrm{Number of neutrons}\\
&=3+4\\
&=7\\
\end{align*}\]
Determine the number of neutrons present in the iodine isotopes, \(\ce{_{53}^{125}I}\) and \(\ce{_{53}^{131}I}\).
Step 1: Find the atomic number (Z) and mass number (A) from the nuclide notation. Z is the number written at the bottom. A is the number at the top.
For \(\ce{_{53}^{125}I}\), \(Z=53\) and \(A=125\).
For \(\ce{_{53}^{131}I}\): \(Z=53\) and \(A=131\).
Step 2: Determine the number of neutrons. This is the mass number (A) minus the atomic number (Z).
For \(\ce{_{53}^{125}I}\):
\[\begin{align*}\textrm{Number of neutrons} & =A-Z\\
& =125-53\\
& =72
\end{align*}\]
For \(\ce{_{53}^{131}I}\):
\[\begin{align*}\textrm{Number of neutrons} & =A-Z\\
& =131-53\\
& =78
\end{align*}\]
Worked example – determining the atomic mass
Chlorine naturally exists in two isotopic forms: \(\ce{_{17}^{35}Cl}\) and \(\ce{_{17}^{37}Cl}\). Each isotope's relative percentage abundance and atomic mass are given in the following table. Calculate the atomic mass of chlorine.
| Isotopes | Relative percentage abundance (%) | Atomic mass (amu) |
|---|---|---|
| Chlorine-\(35\) | \(75.53\) | \(34.97\) |
| Chlorine-\(37\) | \(24.47\) | \(36.97\) |
Step 1: For each isotope, multiply the atomic mass by its percentage abundance.
\[\begin{align*}\textrm{Chlorine-35} & = \textrm{percentage abundance}\times\textrm{atomic mass}\\
& = 75.53\textrm{%}\times34.97\textrm{ amu}\\
& = 26.41\textrm{ amu}
\end{align*}\]
\[\begin{align*}\textrm{Chlorine-37} & = \textrm{percentage abundance}\times\textrm{atomic mass}\\
& = 24.47\textrm{%}\times36.97\textrm{ amu}\\
& = 9.047\textrm{ amu}
\end{align*}\]
Step : Find the sum of the values calculated in Step 1.
\[\begin{align*}\textrm{Atomic mass of chlorine} & = 26.41\textrm{ amu}+9.047\textrm{ amu}\\
& =35.46\textrm{ amu}
\end{align*}\]
Your turn – atomic structure
Test yourself on your understanding of atomic structure.
Images on this page by RMIT, licensed under CC BY-NC 4.0
